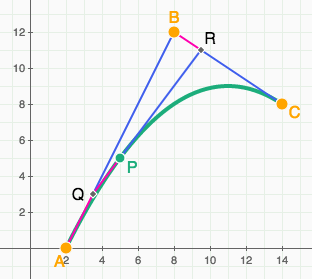
A parabola has been created with a string construction from the control points, $A$, $B$ and $C$. $A = (2, 0)$ $B = (8, 12)$ $C = (14, 8)$ Point $Q$ is on the line $AB$ at $(3.5, 3)$. Point $R$ is on the line $BC$ at $(9.5, 11)$. Line $QR$ touches the parabola at point $P$.  Given that the ratio of $AQ : AB$ equals the ratio of $BR : BC$ what are the coordinates of $P$? $P$ = $($[[☃ math-keypad 1]], [[☃ math-keypad 2]]$)$